- Joined
- Sep 1, 2021
- Messages
- 4
I just wonder the refractive index are the same for both colorless and colored diamond?
I saw all the electronic refractive meter in the market are for colorless diamonds only.
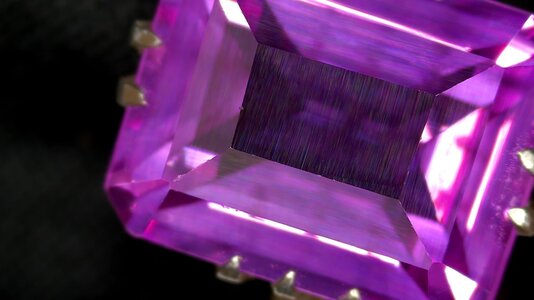
I have a violet/red stone for many years. It is very hard.
It can scratch on ruby.
But RI cannot meet 2.41
I also have a black diamond. the RI is 2.43
I saw all the electronic refractive meter in the market are for colorless diamonds only.
I have a violet/red stone for many years. It is very hard.
It can scratch on ruby.
But RI cannot meet 2.41
I also have a black diamond. the RI is 2.43










300x240.png)