- Joined
- Aug 15, 2000
- Messages
- 18,766
This article http://www.gia.edu/pdfs/Fall_2001_Cut.pdf is a much harder read Rhino, but shows how GIA attempted to quantify or design a metric for fire (DCLR).
Here are some quotes from it:
However, in
the same dictionary, the definition for fire states
merely ¡§see Dispersion.¡¨ Since all diamonds have
the same dispersion value (0.044), this definition is
not adequate. Rather, fire is the result of dispersion.
Thus, we suggest a more direct definition: fire is the
visible extent of light dispersed into spectral colors.
As with WLR to express brilliance, we have developed
a metric, or number, to express how well a
round brilliant can disperse¡Xor spread¡Xlight into
colors (dispersed colored light return, or DCLR, as
defined on p. 181 below).
Our ¡§observer¡¨ for WLR was a hemisphere
at infinity (with a cosine squared weighting
function for the returned light), and we found that
this observer was a good choice for viewing fire
The combination of point light source and infinite
viewing distance yields only dispersed light on
the observing hemisphere; that is, the result appears
as various colored streaks (with no white centers).
These streaks are composed of colored spots showing
the final exit directions of individual rays.
DCLR = „¦streaks „¦colors (Area „ªƒnSmoothed Intensity
„ªƒnWeighting Factor)
The last part is a mathematical formula that probably does not copy and paste.
Sergey showed the GIA researchers after that article, in a never published letter to the Editor of Gems & Gemology, that their formula was independant of dispersion - so they would get the same result for quartzs as for diamond.
It is also clear that they used a model that avoided the white flare that is common in the center of a spectral flash - they did this by using the equivalent of an infinite light surce (like the sun) and assessing the fire at an infinite distance. They wasted a heap of money.
They could have used Sergey''s and Yuri''s free tools that were published years before on the Moscow Uni site and done a better (but still wrong) job.
There is more to be learned from looking at and reading about the images posted here
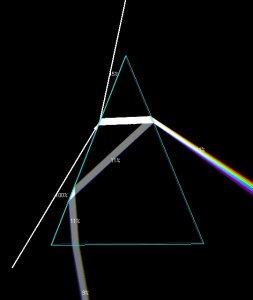
300x240.png)